CryptoDB
Low-Complexity Weak Pseudorandom Functions in AC0[MOD2]
| Authors: |
|
|---|---|
| Download: |
|
| Conference: | CRYPTO 2021 |
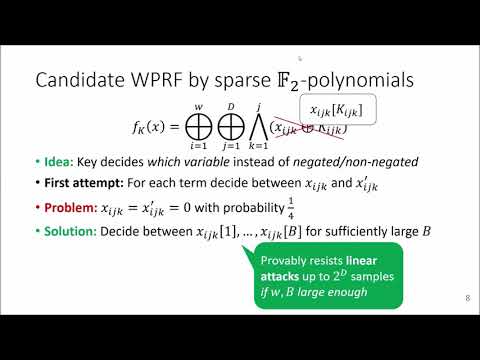
| Abstract: | A *weak pseudorandom function* (WPRF) is a keyed function $f_k:\{0,1\}^n\to\{0,1\}$ such that, for a random key $k$, a collection of samples $(x, f_k(x))$, for {\em uniformly random} inputs $x$, cannot be efficiently distinguished from totally random input-output pairs $(x,y)$. We study WPRFs in AC0[MOD2], the class of functions computable by AC0 circuits with parity gates, making the following contributions. - *Between Lapland and Cryptomania.* We show that WPRFs in AC0[MOD2] imply a variant of the Learning Parity with Noise (LPN) assumption. This gives an unconditional version of an earlier conditional result of Akavia et al. (ITCS 2014). We further show that WPRFs in a subclass of AC0[mod 2] that includes a recent WPRF candidate by Boyle et al. (FOCS 2020) imply, under a seemingly weak additional conjecture, public-key encryption. - *WPRF by sparse polynomials.* We propose the first WPRF candidate that can be computed by sparse multivariate polynomials over $\F_2$. We prove that it has subexponential security against linear and algebraic attacks. - *WPRF in AC0 ◦ MOD2.* We study the existence of WPRFs computed by AC0 circuits \emph{over} parity gates. We propose a modified version of a previous WPRF candidate of Akavia et al., and prove that it resists the algebraic attacks that were used by Bogdanov and Rosen (ECCC 2017) to break the original candidate in quasipolynomial time. We give evidence against the possibility of using {\em public} parity gates and relate this question to other conjectures. |
Video from CRYPTO 2021
BibTeX
@inproceedings{crypto-2021-31253,
title={Low-Complexity Weak Pseudorandom Functions in AC0[MOD2]},
publisher={Springer-Verlag},
doi={10.1007/978-3-030-84259-8_17},
author={Elette Boyle and Geoffroy Couteau and Niv Gilboa and Yuval Ishai and Lisa Kohl and Peter Scholl},
year=2021
}