CryptoDB
Differential Cryptanalysis in the Fixed-Key Model
| Authors: |
|
|---|---|
| Download: | |
| Presentation: | Slides |
| Conference: | CRYPTO 2022 |
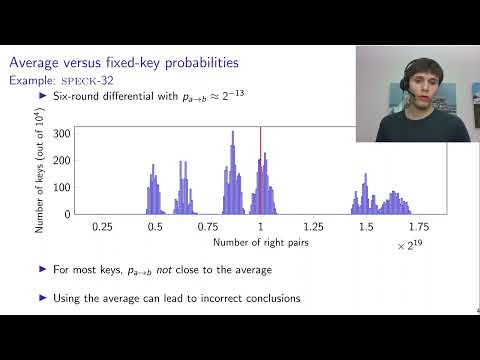
| Abstract: | A systematic approach to the fixed-key analysis of differential probabilities is proposed. It is based on the propagation of 'quasidifferential trails', which keep track of probabilistic linear relations on the values satisfying a differential characteristic in a theoretically sound way. It is shown that the fixed-key probability of a differential can be expressed as the sum of the correlations of its quasidifferential trails. The theoretical foundations of the method are based on an extension of the difference-distribution table, which we call the quasidifferential transition matrix. The role of these matrices is analogous to that of correlation matrices in linear cryptanalysis. This puts the theory of differential and linear cryptanalysis on an equal footing. The practical applicability of the proposed methodology is demonstrated by analyzing several differentials for RECTANGLE, KNOT, Speck and Simon. The analysis is automated and applicable to other SPN and ARX designs. Several attacks are shown to be invalid, most others turn out to work only for some keys but can be improved for weak-keys. |
Video from CRYPTO 2022
BibTeX
@inproceedings{crypto-2022-32245,
title={Differential Cryptanalysis in the Fixed-Key Model},
publisher={Springer-Verlag},
author={Tim Beyne and Vincent Rijmen},
year=2022
}